Устойчивость конструкции - явление не новое при расчете стальных конструкций. Канадская норма для расчета стали CSA S16 и ее последняя версия 2019 года не являются исключением. Подробные требования к устойчивости могут быть выполнены либо с помощью упрощённого метода расчёта на устойчивость, описанного в разделе 8.4.3, либо, согласно новой версии 2019 г., с помощью метода эффектов устойчивости в упругом расчёте, указанном в приложении O [1].
Clause 8.4.1 [1] lists the stability requirements which the structural design should address using either method. These include deformations contributing to the structure, second order effects including P-Δ and P-δ, global and member geometric imperfections, stiffness reduction accounting for member yielding and residual stresses, and lastly, uncertainty in the structure’s stiffness and strength.
Clause 8.4.3 – Simplified Stability Analysis Method
With the simplified stability analysis method given in 8.4.3 [1], only a couple requirements are listed.
Геометрические нелинейности
Первое включает в себя эффекты второго порядка у стержней или P-Δ, которые можно учесть непосредственно при расчете. В настоящее время анализ второго порядка для вычислений используется в большинстве программ для расчета конструкций. The alternative is to amplify all member axial loads and bending moments obtained from a first-order analysis by the factor U2 defined in 8.4.3.2(b) [1]. Данный порядок больше подходит для ручных расчетов или в тех случаях, когда программное обеспечение для расчета конструкций не учитывает автоматически эффекты P-Δ.
Геометрические несовершенства
Notional lateral loads are the second item listed under the simplified method in Clause 8.4.3.3 [1]. Приложенная нагрузка соответствует 0,005-кратной общей расчетной нагрузке от собственного веса на рассчитываемом этаже и должна распределяться аналогично весовой нагрузке. Условные нагрузки всегда действуют в том направлении, в котором вызовут наибольший дестабилизирующий эффект. Это означает, что данные нагрузки должны быть приложены в том же направлении, что и боковая ветровая нагрузка, для того, чтобы вызвать наибольшие деформации и внутренние силы в конструкции.
Annex O.2 – Stability Effects in Elastic Analysis
As an alternative to the simplified stability analysis approach above, engineers can utilize Annex O.2 to satisfy the stability requirements set forth in Clause 8.4.1 [1]. Данный подход был включен в норму в 2019 году и имеет много общего с американской нормой по стальным конструкциям AISC 360-16, глава C Прямой метод расчета.
Геометрические нелинейности
Geometric nonlinearities, or second-order effects, are addressed in O.2.2 [1]. Like the simplified method, a second-order analysis can be carried out directly which includes the effects of loads acting at members’ displaced points of intersections (P-Δ effects). Кроме того, необходимо учитывать влияние осевых нагрузок, действующих на изогнутый по длине стержень (P-δ). There are provisions given in O.2.2 [1] where P-δ can be neglected entirely. On the other hand, if P-δ is included directly in the analysis, the factor U1 can be set to 1.0 used in Clause 13.8 - Axial compression and bending member design [1].
Геометрические несовершенства
Member geometric imperfections such as member out-of-straightness or local geometric imperfections such as element out-of-straightness for members do not need to be considered when designing to Clause O.2 [1]. При этом глобальные геометрические несовершенства необходимо учитывать в прямом моделировании или при применении условных боковых нагрузок. There is the exception though that these global geometric imperfections can be neglected for lateral load combinations only if they meet the requirements set forth in Clause O.2.3.1 [1]. The requirements include the structure’s gravity loads are supported primarily by vertical structural elements and the ratio between maximum 2nd order story drift to 1st order story drift using reduced member stiffness according to Clause O.2.4 [1] does not exceed 1.7 at any story level.
Если данными несовершенствами нельзя пренебречь, то можно применить первый метод прямого моделирования. Точки пересечения стержней должны быть перемещены с исходных положений. The amplitude of this initial displacement is set forth in Clause 29.3 [1] and applied in the greatest destabilizing direction, which for most building structures is a 1/500 tolerance for column out-of-plumbness. Основная проблема данного метода заключается в большом количестве модельных сценариев, которые необходимо рассмотреть. Теоретически на каждом уровне этажа требуется четыре перемещения в четырех различных направлениях. Если эффекты непрямолинейности стержня сочетаются с отклонением от вертикали колонны, то возникает намного больше модельных сценариев для достижения наибольшего дестабилизирующего эффекта.
Альтернативным предпочтительным методом при глобальных геометрических несовершенствах является применение условных боковых нагрузок. Применение этого метода допускается только в том случае, если нагрузки от собственного веса воспринимаются главным образом вертикальными конструктивными элементами. Notional lateral loads were covered earlier in this article and are applied in the same manner as the simplified stability analysis in Clause 8.4.3.2 [1]. Однако предел на соответствующем этаже уменьшается с 0,005 до 0,002-кратной расчетной нагрузки от собственного веса. The reduction in magnitude is permitted in Clause O.2.3.3 as these notional loads account for global geometric imperfections only, whereas notional loads in Clause 8.4.3.2 [1] are also accounting for inelasticity effects and other uncertainties.
Эффекты неупругого деформирования
To account for inelasticity effects and to also give consideration for initial member or local geometric imperfections as well as uncertainty in stiffness and strength, reduced member axial and flexural stiffness according to the following equations in Clause O.2.4 [1] should be applied to members contributing to the lateral stability.
- EAr = 0.8τbEA
- EIr = 0.8τbEI
Где:
- Cf/Cy < 0.5 ; τb = 1.0
- Cf/Cy > 0.5 ; τb = 4Cf/Cy(1-Cf/Cy)
Во избежание локализованных деформаций, норма предлагает применить данное уменьшение жесткости ко всем стержням. Кроме того, когда жесткость при сдвиге (GA) и жесткость при кручении (GJ) в значительной мере обеспечивают боковую устойчивость, следует рассмотреть возможность снижения жесткости. Снижение жесткости нельзя применять при расчете смещений, прогибов, колебаний или собственных колебаний.
Annex O.2 Application in RFEM 6
The new generation FEA program RFEM 6 incorporates the latest stability requirements of the CSA S16:19 standard according to the Annex O.2 provisions.
Геометрические нелинейности
Second-order effects set forth in Clause O.2.2 [1] are directly considered when the static analysis calculation method is set to “Second-order (P-Δ)”. This can be applied within the Design Situation Combination Wizard options. In turn, all load combinations under the Design Situation will automatically be set to a second-order analysis as well. The user has the option to individually modify a load combination’s static analysis settings if preferred.
Not only are P-Δ effects included for the member analysis, but P-δ are also automatically considered. For more information on this topic and verification in RFEM 6, take a look at: База знаний 1759 .
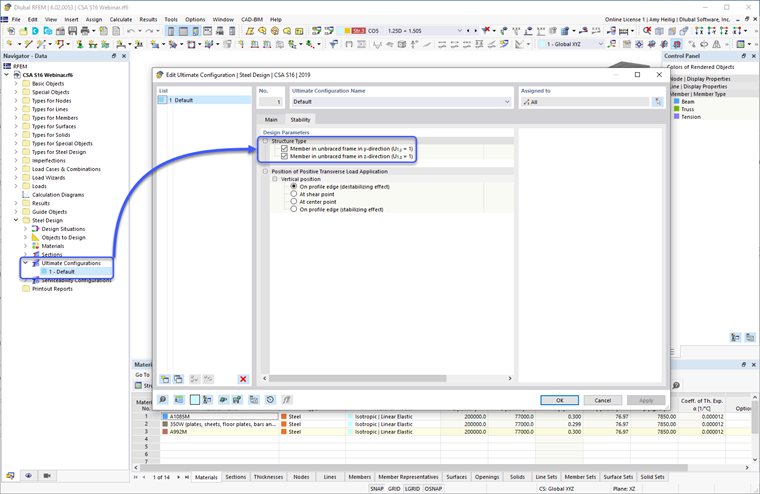
Therefore, the factor U1 can be set to 1.0 specified in Clause 13.8 for the steel member design. This option is found under the Steel Design Add-on – Ultimate Configurations – Stability – Design Parameters.
Геометрические несовершенства
The RFEM 6 user has the option to directly model global geometric imperfections by displacing points or nodes of member intersections. Однако, чтобы гарантировать, что данный метод создаст наибольший дестабилизирующий эффект, необходимо будет выполнить несколько моделей с различными сценариями. Это довольно затратно по времени и трудоемко.
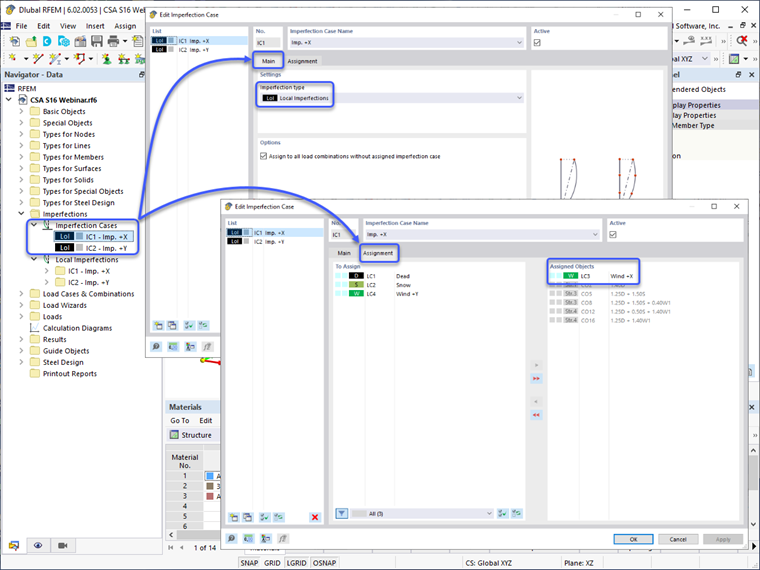
The alternative approach is to apply notional loads with the imperfection options provided in RFEM 6. To begin, Imperfection Cases with the imperfection type set to “Local imperfections” under the Main tab must first be defined. These would typically include cases in the orthogonal directions X and Y depending on the application of lateral loads such as wind and seismic. The Imperfection Case can then be correlated under the Assignment tab to the specific load cases to produce the greatest destabilizing effect (for example, notional loads in the +X-direction should only be applied with wind loads in the +X-direction).
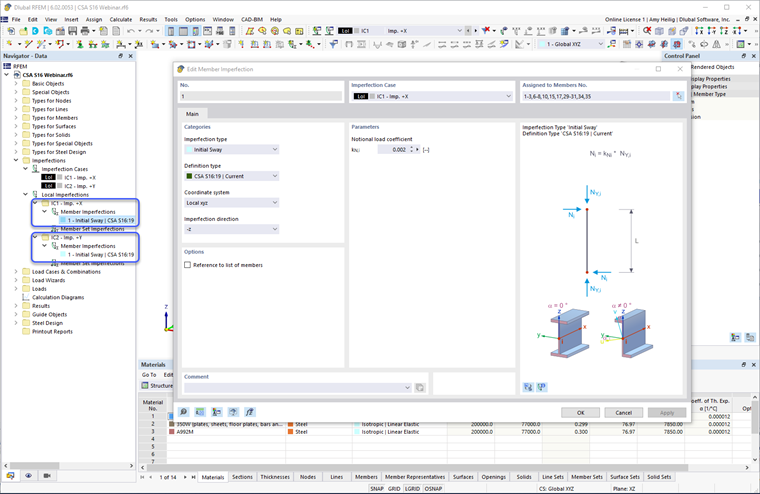
After the Imperfection Cases are generated, the member imperfections can be defined. The Member Imperfections dialog box includes the CSA S16:19 in the dropdown options. The notional load is applied to the member end (i.e., top of column) with a magnitude equal to 0.002 (or 0.005 if using the simplified stability method) multiplied by the member’s axial force (applied member gravity load). An equal and opposite force is internally applied at the opposite member end to avoid unrealistic base shears. The imperfection definition is applied to the members’ local axes in the same direction as the applied lateral load such as wind or seismic. The definition is further applied to all vertical members in the model.
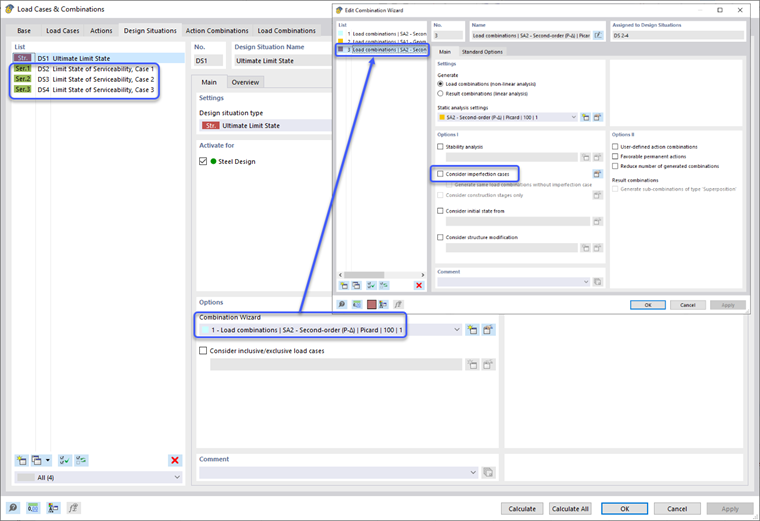
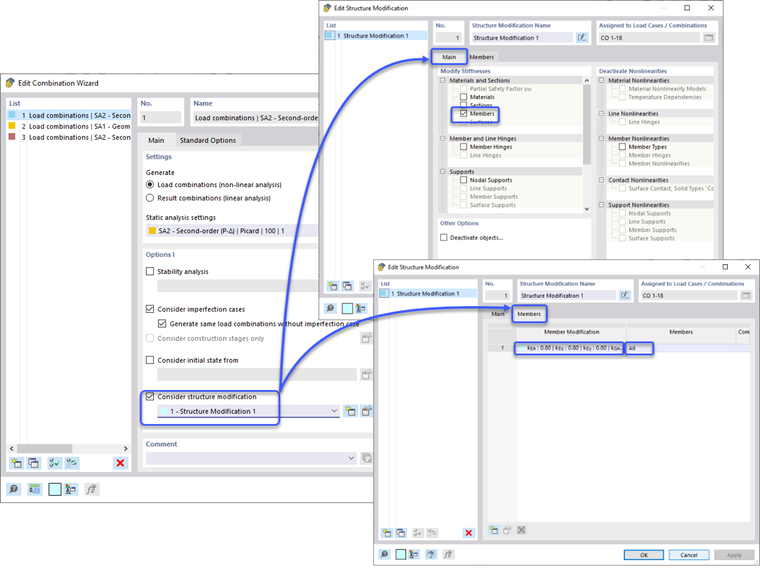
After the imperfections are applied to the model, the Design Situation is set by default to consider imperfections for all load combinations. Imperfections should be applied to the Ultimate Design Situations but turned off for the Serviceability Design Situations. This can be set by creating a new Combination Wizard definition type, turning off the imperfection consideration options, and applying to the Serviceability Design Situations only.
Эффекты неупругого деформирования
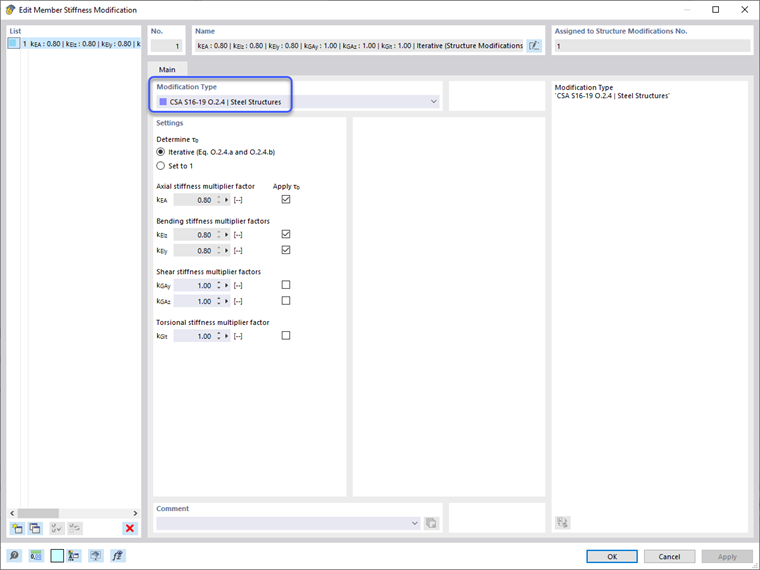
Stiffness reductions are applied to the Ultimate Design Situation only once again through the Combination Wizard definition options and the “Consider structure modification” checkbox. A new structure modification definition can be created. The “Members” checkbox is selected under the Main tab - Materials and Sections. This brings up a new Members tab where the Member Stiffness Modification definition is defined according to the CSA S1-19 O.2.4 | Steel Structures. This definition type allows the program to either calculate the τb reduction factor automatically or a generalized 1.0 value can be set for all members. Additionally, the 0.8 factor can be applied to the various member stiffness types. The user can determine if the τb and 0.8 factor should be applied only to the member axial and bending stiffness or if shear and torsional stiffness should also be considered. Once the stiffness modification properties are input, the definition can be applied to specific members or the term “All” can be set to apply to all members in the model.
Because member stiffness reduction should not be considered for serviceability design (for example, deflection checks), the “Consider structure modification” checkbox should remain unchecked for the Serviceability Design Situation Combination Wizard definition.
After these modifications, all factored load combinations will include the structure modification stiffness reduction while all unfactored load combinations will use the full member stiffness.
Заключение
Significant stability design requirements according to Annex O.2 in the Canadian steel design manual CSA S16:19 are fully incorporated in the RFEM 6 analysis workflow. Most notably, these requirements include a second-order analysis, the ability to consider notional loads as imperfections, as well as reduced member stiffnesses. To see this topic demonstrated in a detailed example video, check out the webinar: Расчет стальных конструкций по норме CSA S16:19 в программе RFEM 6 (США) .