输入壳模型
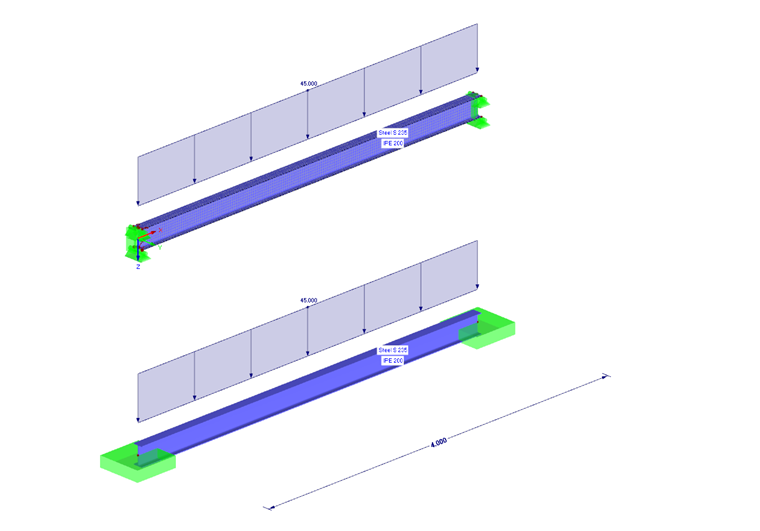
在RFEM中创建壳模型非常简单。 可以选择直接在面中生成杆件单元(“由杆件生成面”功能)。 首先创建杆件的长度为4 m。 截面类型为IPE 200。 在对梁进行建模之后,可以使用上述功能在杆件中生成面。
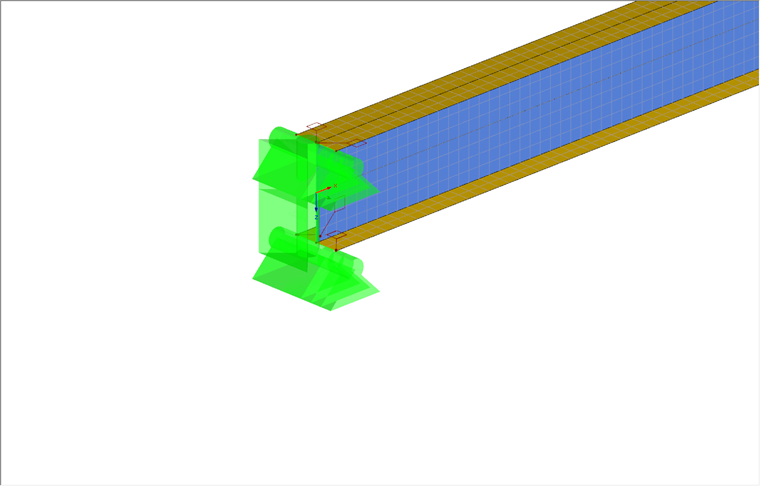
在建立梁的纯壳模型之后,您可以定义边界条件。 梁的两侧都应该被支撑。 这些边界条件可以通过线支座来创建。 在该应用中,面模型的腹板和翼缘可以通过线支座进行支撑。 Eine komplette Einspannung der Lager ist dabei nicht nötig, da sich die Einspannung aus der Begrenzung der Wegfreiheitsgrade an Steg und Flansch ergibt.
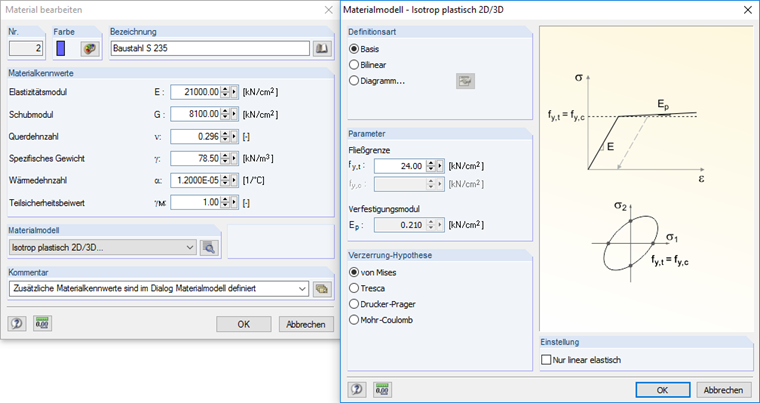
输入边界条件后,您可以通过选择各向同性塑性2D/3D材料模型来定义面的塑性行为。 该材料模型可以在计算过程中考虑面的塑性。 当材料的屈服强度设为24 kN/cm²时,也可以在同一对话框中设置von Mises等效应力。 当您指定材料的塑性行为时,在计算参数中的荷载增量将自动激活。 荷载增量将有助于更有效的收敛行为。
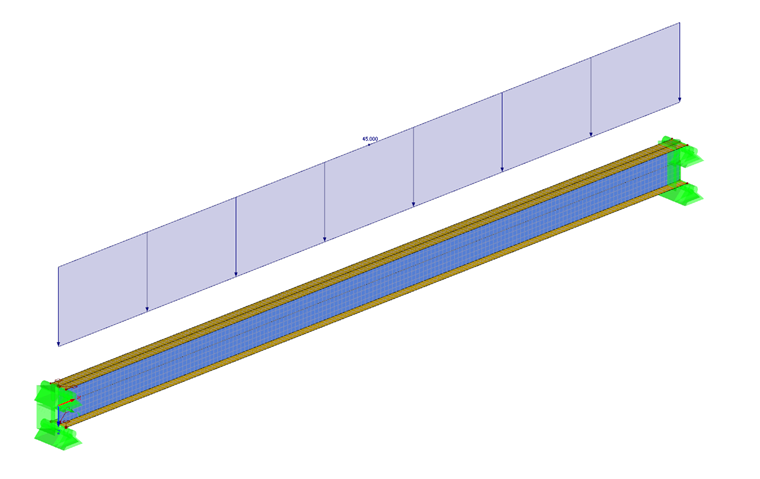
在上部翼缘和腹板的交点处,线荷载作用在结构上。 荷载大小为45 kN/m,因为在两个支承区域开始形成塑性铰。
变形在计算整个结构后立即显示。 根据von Mises可以将视图切换为等效应力。 RFEM的默认设置显示带有平滑轮廓的应力。 这会导致结果的变形,因为超过了塑性最大应力。 因此,面上的内力和应力必须选择“单元上的常量”显示选项。 这些结果表示每个有限元单元的平均值。 使用有限元单元的节点值生成平均值。 当使用塑性或非线性材料属性时,始终选择“单元中的常数”显示选项,因为这种材料属性会影响单元值的塑性,从而正确显示塑性应力。
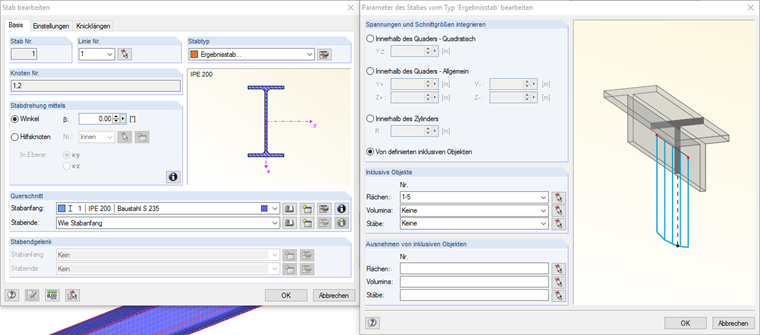
为了与分析计算进行比较,必须使面模型的结果与分析模型的结果可比较。 为此可以使用结果梁。 使用结果梁可以将模型中的所有面应力或实体应力集成在一起。 进一步与分析模型进行比较。
在模型中定义杆件非常简单。 在将一维杆件生成面模型时,在原始杆件的位置出现一个虚拟杆件,该杆件用作占位符。 该杆件没有刚度,在计算中将不考虑。 杆件类型从“虚拟”更改为“结果梁”,然后将所有面分配到该结果梁上作为一个合力值查看内力。 在本例中,翼缘面和腹板面包含在结果梁中,可以将单元的内力结果视为单个杆件。
Eingabe des Stabmodells
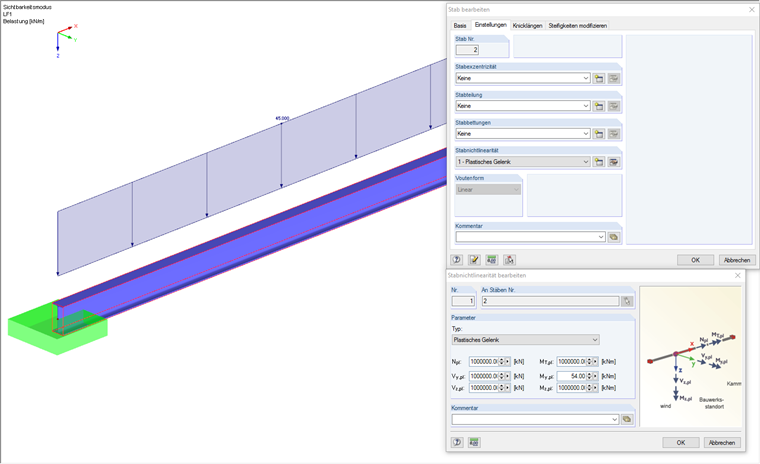
为了进行比较,现在创建一个简单的杆件模型并加载以形成一个塑料铰链。 定义了一个截面为IPE 200的简单杆件。 对于该杆件,我们将创建具有各向同性材料属性的附加材料。 为此钢结构选择了钢类型为S235。 在杆件非线性下还可以考虑考虑塑性铰。 由于只定义了塑性力矩的释放,因此所有其他的内力都设置为一个较大的值,因此不会受到影响。 使用S235可以计算IPE 200的塑性极限弯矩:
M层 = fy ∙W层
Mply = 24 kN/cm 2 ∙220.6 cm 3 = 54 kNm
Die Randbedingungen werden als beidseitig eingespannt angenommen, um mit dem Flächenmodell vergleichbar zu bleiben. 该荷载在本示例中作为杆件荷载应用,因为线荷载只能用于面。 最大杆件荷载为45 kN/m。
计算分析计算
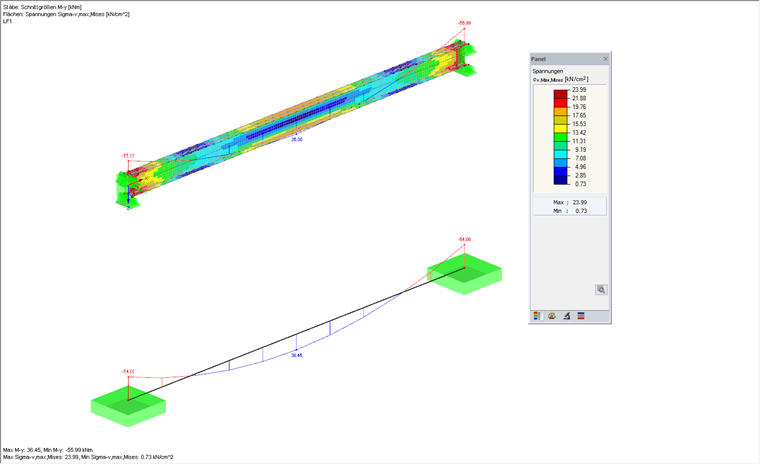
两种计算的结果都可以在下图中进行比较。 结果几乎相同。 使用面模型可以清楚地看到在支座上的塑性铰。 作用在结果梁上的内力与包含塑性铰的杆件模型的内力非常相似。 结果差异可以归因于面模型的建模和杆件模型的理想化。