结果组合2 | 应用示例和荷载组合比较

示例

可用的荷载工况有以下几种：
LC1 - 自重
LC2 - 雪荷载
LC3 - 活荷载 - 方案 1
LC4 - 活荷载 - 方案 2

我们正在按照 EN 1990 查找承载能力极限状态 (ULS) 的内力包络线。 在组合中可以考虑施加的荷载工况。

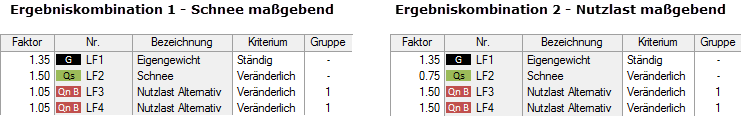
通过结果组合进行组合

按照 EN 1990，式6.10, 作用应提供分项安全系数和组合系数。 但是，在定义结果组合时，您只能指定一个系数。 因此，如果不清楚哪个可变作用是主导的可变作用，则应该创建多个作用组合。

结果组合

在分配了系数之后，您应该定义一个准则和一个组。 “自重”内力应始终适用，因此定义为“永久”内力。 可能会出现其他荷载工况，但不是必须的。 因此在这种情况下分配给“可变”的准则。 交替作用的施加荷载工况的细节由该组控制。 两个强制荷载工况的编号相同。 因此在该组合中只使用一个强制荷载工况。

生成的最终包络结果组合如下：
RC3 (ULS) = RC1/s 或 RC2/s

根据这个理论示例，RC1 和 RC2 有以下内力，RC3 有以下内力计算公式：

包络

通过荷载组合进行组合

现在将通过荷载组合对相同的四种荷载工况进行叠加。 为了确保找到最不利的内力，会创建所有可能的内力组合。 计算时将考虑主要的可变荷载以及施加荷载的替代作用，生成这些效应。 后续与结果组合进行比较的基本要求是使用线性系统，并且按照线性静力分析进行荷载组合的计算。

组合的分类

总共需要 8 种荷载组合选项。 为了将结果与结果组合进行比较，创建另一个包络 RC，其中包括荷载组合的结果：
RC4 = CO1/p 或 CO2/p 或 CO3/p 或 CO4/p 或 CO5/p 或 CO6/p 或 CO7/p 或 CO8/p

基于相同的系统，我们得到以下内力：

RC4的结果

结果比较

如果你比较 RC3 和 RC4 的内力，那么在这个例子中没有发现差异。 值得注意的是，在使用荷载组合的求解中总共需要创建 9 个组合（8 个 LC + 1 个 RC），而使用结果组合的求解只需要 3 个组合。 这种节省会对计算时间以及在程序尤其是报告中的概览产生积极影响。

小结

在几何线性模型中可以按照一阶理论进行计算，结果组合是组合荷载工况的有效工具。 这在分析具有大量荷载工况（例如运动荷载）的结构时尤其有用，否则会导致大量的荷载组合，从而导致记录不清楚。