Ответ:
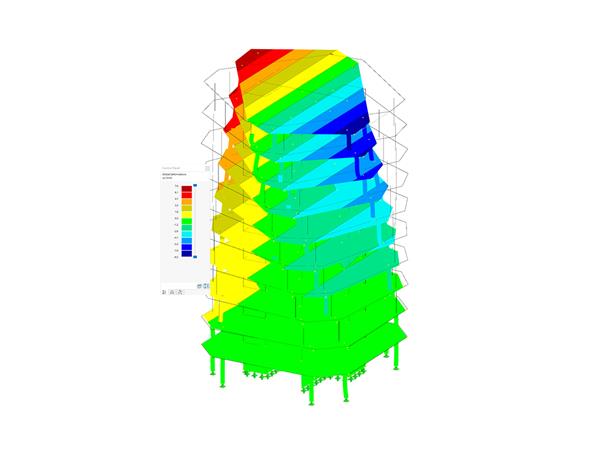
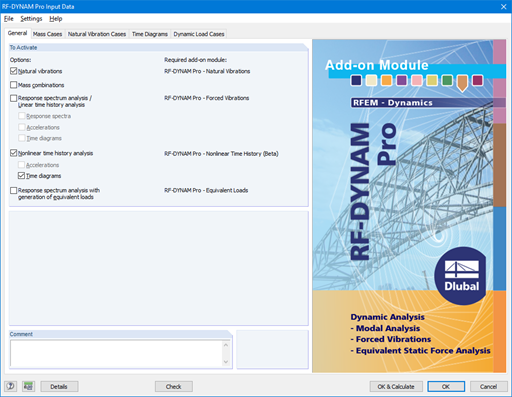
Модуль RF-DYNAM Pro - Nonlinear Time History содержит в себе как неявный решатель метода среднего ускорения (по методу среднего ускорения), так и явный решатель.
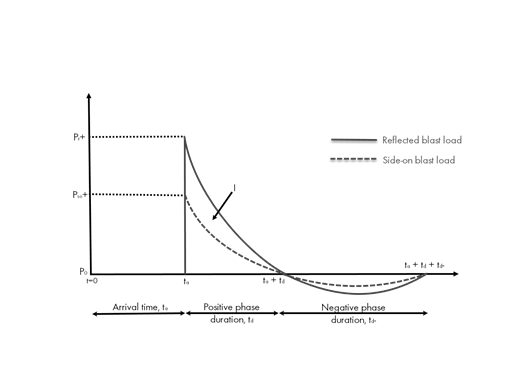
Hierbei ist zu beachten, dass nicht die „originale" Variante des Zentralen Differenzenverfahren verwendet wird sondern eine abgewandelte Form. Die Abwandlung ist dadurch charakterisiert, dass es sich beim Ansatz der Geschwindigkeitsdifferenz eben nicht um eine zentrale Differenz handelt. Die nachstehenden zwei Gleichungen zeigen die angesetzten Differenzen der Geschwindigkeit und der Beschleunigung:
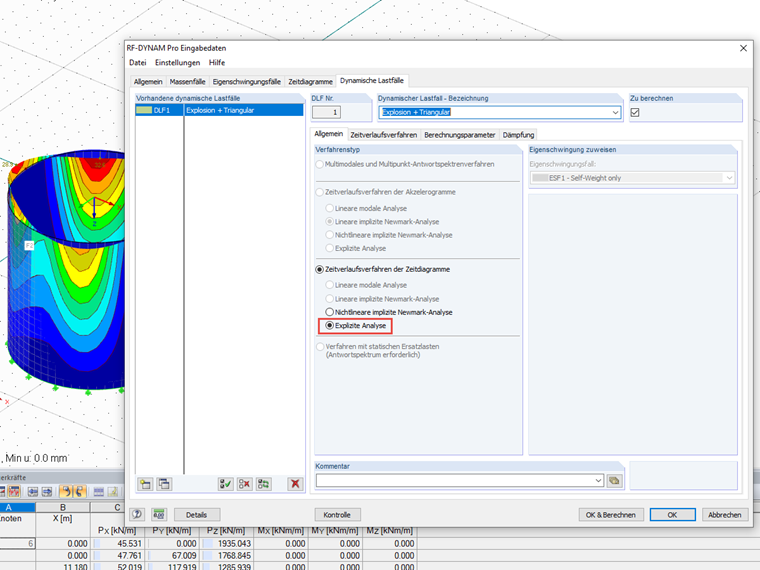
Dieser Ansatz führt zu einer schnelleren Konvergenz, da „schneller“ auf Änderungen in der Belastung bzw. auf Systemänderungen (Nichtlinearitäten) reagiert wird. Wann die explizite Analyse gewählt werden sollte, ist in FAQ 2356 ausführlich beschrieben.
