结果组合 | 1. 基本

定义的依据

使用结果组合对荷载工况进行组合，可以对结果进行简单的添加和比较（“或”）。 因此，结果组合的定义比荷载组合的定义更复杂，并且主要取决于“系数”，“ Criterion”和“ Group”。

01 – 结果组合

如果选择“系数”，则是荷载工况的乘积。 通常在这里定义分项系数或组合系数。 正值表示加法，负值表示减法。

“标准”确定荷载工况的结果是始终应用组合（“永久”）还是仅具有偶然作用（“变量”）。 因此，如果荷载工况的结果对结果有不利的影响，那么在计算结果中只考虑“ Variable”准则的结果。 由于尚不清楚最大的正值还是最大的负值会导致不利的结果，因此记录两个值。

使用“组”，您可以选择荷载工况的替代作用。 例如，如果将两个荷载工况分配到同一组，那么在组合中考虑第一个或第二个荷载工况的结果。

在下面的两个例子中，结果组合被用于结果的叠加（示例1），以及用于寻找和选择作用的多个荷载工况的最大和最小值（示例2）。

示例1： 结果相加

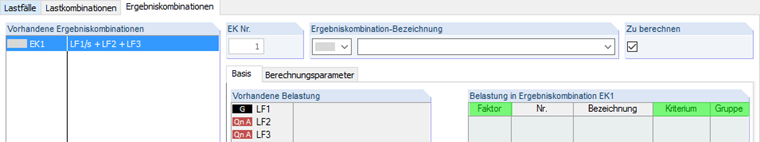
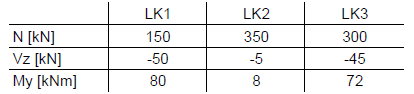
在模型中相同的x位置上有3个不同的荷载工况的结果。 仅简化地考虑了法向力N，剪力V_z和弯矩M_y 。 荷载工况1包含永久荷载，其他两个荷载工况为强加荷载工况。

02 – 内力（按荷载工况）

使用结果组合，将所有三个荷载工况叠加在一起。 荷载工况定义如下：

3 - Addition mittels Ergebniskombination

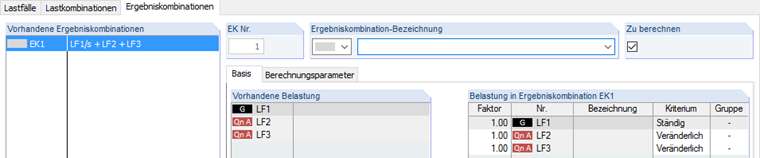
由于荷载工况1包含永久荷载，因此将“永久”条件分配给该荷载工况。 施加的荷载工况可以起作用，但不是必须的。 因此，该标准设置为“变量”。 此外，两个施加的荷载工况可能同时发生。 因此，该组未被定义。 为了简化理论计算，不使用分项系数（factor = 1.0）。

由此得出每个内力的最大值和最小值。 同样显示相应的内力。

4 - 结果组合

让我们在第一行中计算最大N值时更详细地说明结果。 在这种情况下，计算的依据是荷载工况1，因为它被定义为“永久”作用。 其他两个荷载工况的法向力只有在它们增加了法向力时才使用。 荷载工况2增加最大法向力，而荷载工况3再次减小。 因此，只有荷载工况1和荷载工况2施加于最大法向力：
最大N = -20 + 200 = 180 kN

相关的内力必须使用相同的组合计算：
关系 V_z = 25 + 5 = 30 kN
关系 M_y = -50 +（-10）= -60 kNm

以同样的方式计算下面的线，得出每个内力的最大值和最小值，包括相关值。 现在可以将其用于进一步的设计。

示例2： 荷载作用的结果包络以及其他作用

在第二个示例中计算三个荷载组合的结果。 当然不能将它们组合在一起，但是可以将交替作用的部分相互比较。 目的是按照与第一个示例类似的方法获得最大和最小作用力。 可以使用以下值：

05 - 内力（按荷载工况）

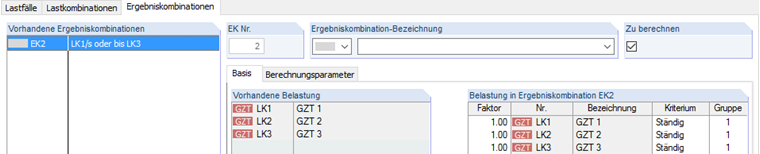
结果组合定义如下：

06 – 结果组合作为选替作用的荷载情况包络

荷载组合的结果已经包含了几乎所有的部分安全系数和组合系数。 因此该系数保持为1.0。 为了进行备选分析，每个荷载组合必须具有相同的组编号。 这样可以记录CO1，CO2或CO3的结果。 同样重要的是给所有组合指定“永久性”标准。 也就是说，必须始终使用三个组合的结果。 如果对所有组合都指定了“永久”规范，则最小法向力不是150 kN，而是0 kN。 在这种情况下，如果三个荷载组合都不起作用，则法向力为零。 下表中显示了最大和最小内力：

07 – 结果组合的结果

为了清楚起见，将再次说明第一行。 我们正在寻找最大法向力。 在二氧化碳中的含量为350 kN。 计算V_z和M_y时，仅需采用CO2中相应的内力。 因此，所得的V_z为-5 kN，M_y为8 kNm。 这些结果可以用于附加模块的设计。

概述总结

本文介绍定义结果组合的基础，并举例说明最常见的应用。 当然也可以将这些定义过程结合起来。 这将在本系列文章的第2部分中与荷载组合的比较一起进行说明。

参考