8135x

001450

Sonja von Bloh, M.Sc.

2017-06-12

Loads on Silo Hopper According to EN 1991-4

The previous article described the actions on silos according to DIN EN 1991-4. On an example of a free standing cylindrical silo for cement with a conical hopper, filling loads of the silo hopper were calculated.

Layout and Dimensions

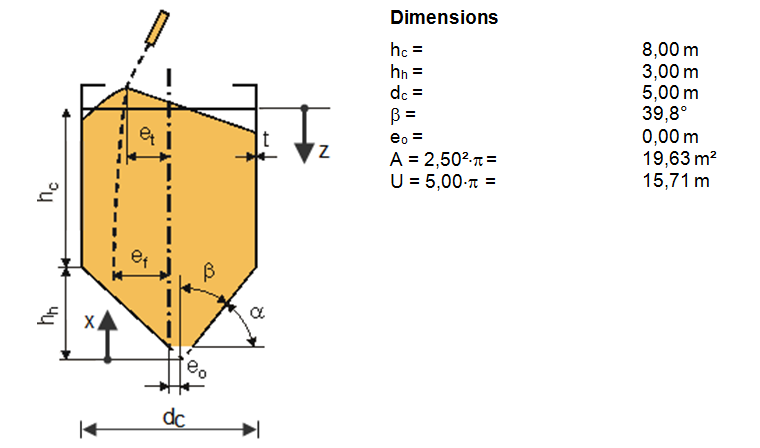
The structural system is shown in Image 01.

System and Component Dimensions of Cement Silo

Governing characteristic values for different load applications

The applicable extreme values of particulate solids for the maximum hopper pressures in the full condition are included in the following table.

Relevant Parameters for Different Load Applications

Physical properties

The loads on the walls of silo hoppers should be determined according to EN 1991‑4 [1], 6.1.1(2) with regard to the steepness of the hopper walls in compliance with the following classes:

A flat bottom shall have an inclination to the horizontal α less than 5°.

A shallow hopper shall be any hopper not classified as either flat or steep.

A steep hopper shall be any hopper that satisfies the following criterion:

\tan β < \frac{1 - K}{2 \cdot μ_{h}} (6.1)

Formula 1

\tan 39.8 ° = 0.83 > \frac{1 - 0.450}{2 \cdot 0.458} = 0.60

Formula 2

The hopper is classified as a shallow hopper.

Filling Loads

Janssen characteristic depth z_o

z_{o} = \frac{1}{K \cdot μ} \cdot \frac{A}{U} (5.75)

Formula 3

z_{o} = \frac{1}{0.450 \cdot 0.458} \cdot \frac{19.63}{15.71} = 6.07 m

Formula 4

Vertical distance h_o
For a symmetrically filled circular silo, the vertical distance h_o between the equivalent surface of the solid and the highest solid‑wall contact is calculated as follows:

h_{o} = \frac{d_{c}}{6} \cdot \tan ϕ_{r} (5.78)

Formula 5

h_{o} = \frac{5.00}{6} \cdot \tan 36.00 ° = 0.61 m

Formula 6

Parameter n

n = - (1 \tan ϕ_{r}) \cdot (\frac{1 - h_{o}}{z_{o}}) (5.76)

Formula 7

n = - (1 \tan 36.00 °) \cdot (\frac{1 - 0.61}{6.07}) = - 1.55

Formula 8

Coordinate z
z = h_c = 8.00 m 6.1.2(2)

Vertical pressure p_vf

p_{vf} = γ \cdot (h_{o} - \frac{1}{n 1} \cdot (z_{o} - h_{o} - \frac{(z z_{o} - 2 \cdot h_{o})^{n 1}}{(z_{o} - h_{o})^{n}}) (5.79)

Formula 9

p_{vf} = 16.00 \cdot (0.61 - \frac{1}{- 1.55 + 1} \cdot (6.07 - 0.61 - \frac{(8.00 + 6.07 - 2 \cdot 0.61)^{- 1.55 + 1}}{(6.07 - 0.61)^{- 1.55}}) = 69.27 kN / m ²

Formula 10

Bottom load magnifier C_b
C_b = 1.0 (6.3)
The bottom load magnifying factor C_b applies to silos of Action Assessment Class 2 under the condition that the stored solids do not tend to dynamic behavior.

Mean vertical pressure at the hopper transition
p_vtf = C_b · p_vf (6.2)
p_vtf = 1.0 · 69.27 = 69.27 kN/m²

Mobilized friction
In a shallow hopper, the waIl friction is not fully mobilized. The mobilized or effective wall friction coefficient should be determined as:

μ_{heff} = \frac{1 - K}{2 \cdot \tan β} (6.26)

Formula 11

μ_{heff} = \frac{1 - 0.450}{2 \cdot \tan 39.8 °} = 0.33

Formula 12

Parameter n
n = S · (1 - b) · μ_heff · cot β (6.28)
S = 2 (6.9)
n = 2 · (1 - 0.2) · 0.33 · cot 39.8° = 0.634

Parameter F_f

F_{f} = 1 - \frac{b}{\frac{1 \tan β}{μ_{heff}}} (6.27)

Formula 13

F_{f} = 1 - \frac{0.2}{\frac{1 \tan 39.8 °}{0.33}} = 0.943

Formula 14

Parameter n
n = S · (F_f · μ_heff · cot β + F) - 2 (6.8)
n = 2 · (0.943 · 0.33 · cot 39.8° + 0.943) - 2 = 0.634

Loads perpendicular to hopper walls
p_nf(x) = F_f · p_v(x) (6.29)

p_{nf} (x) = F_{f} \cdot (γ \cdot \frac{h_{h}}{n - 1} \cdot (\frac{x}{h_{h}} - (\frac{x}{h_{h}})^{n}) p_{vft} \cdot (\frac{x}{h_{h}})^{n}

Formula 15

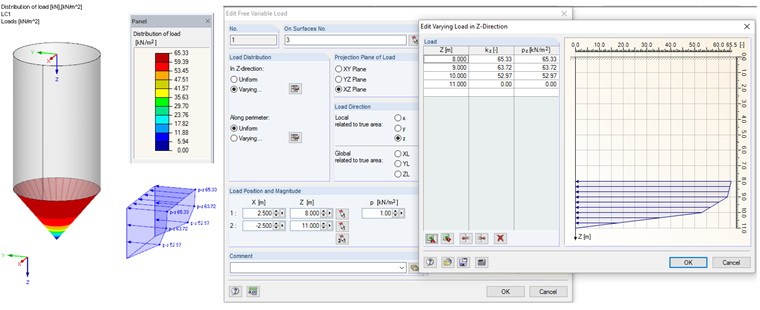
p_nf(0.00) = 0.00 kN/m²
p_nf(1.00) = 52.97 kN/m²
p_nf(2.00) = 63.72 kN/m²
p_nf(3.00) = 65.33 kN/m²

This load can be entered in RFEM as a free variable load. The load input is displayed in Image 03.

Loads Perpendicular to Hopper Walls pnf

Hopper frictional traction
p_tf(x) = μ_heff · F_f · p_v(x) (6.30)
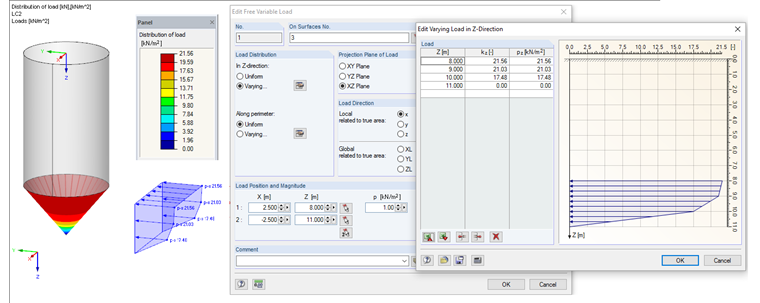
p_tf(0.00) = 0.00 kN/m²
p_tf(1.00) = 0.33 · 52.97 = 17.48 kN/m²
p_tf(2.00) = 0.33 · 63.72 = 21.03 kN/m²
p_tf(3.00) = 0.33 · 65.33 = 21.56 kN/m²

This load can be entered in RFEM as a free variable load. The load input is displayed in Image 04.

Frictional Loads in Hopper ptf

References

[1] EC 1. (2012). Eurocode 1: Actions on Structures – Part 4: Silos and Tanks, EN 1991‑4:2010‑12.

Author

Ms. von Bloh provides technical support for our customers and is responsible for the development of the SHAPE‑THIN program as well as steel and aluminum structures.

Links

Analysis & Design Software for Silos and Storage Tanks

Downloads

RFEM Model File

8.48 MB

;