Distortional Buckling Design of Bottom Flange of Steel Frame Beam According to GB

Technical Article

New

05/30/2023

001824

Steel Design for RFEM 6

RSTAB 9

Steel Design for RSTAB 9

Steel Structures

Structural Analysis

GB 50017

When a concrete slab is set upon the top flange, its effect is like a lateral support (composite construction), preventing problems of torsional buckling stability. If there is a negative distribution of the bending moment, the bottom flange is subjected to compression and the top flange is under tension. If the lateral support given by the stiffness of the web is insufficient, the angle between the bottom flange and the web intersection line is variable in this case so that there is a possibility of distortional buckling for the bottom flange.

0. Overview

The Chinese steel construction standard GB 50017 [1] describes the calculation method for designing the distortional buckling of a frame beam's bottom flange in Chapter 6.2.7. In contrast to the previous standard, this section represents a new added design check.

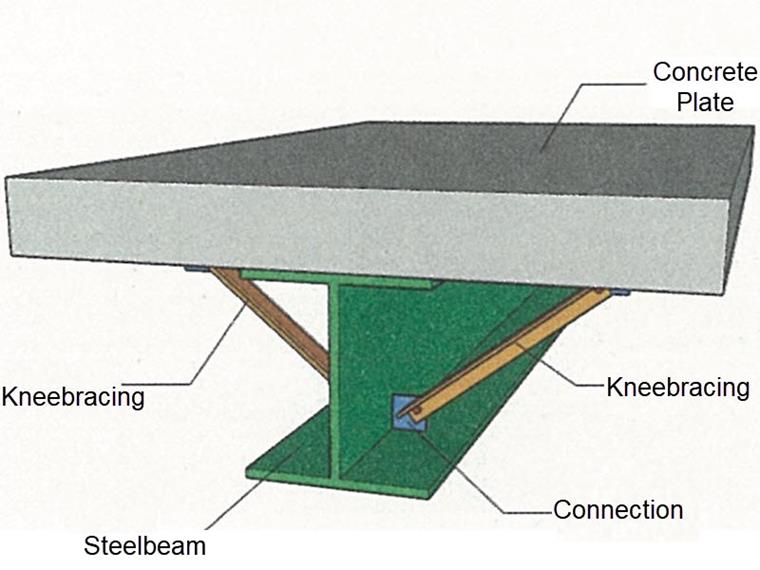
The chapter is related to the stability calculation of the negative moment section of a frame beam. It provides the basis for verifying the stability of the beam's bottom flange or whether structural measures are to be taken.

While the top flange is supported, the bottom flange being subjected to compression can laterally deflect. If the lateral support given by the stiffness of the web is insufficient, the angle between the bottom flange and the web intersection line is variable in this case so that there is a possibility of distortional buckling for the bottom flange.

Laterally Supported Bottom Flange Under Compression

Laterally Supported Bottom Flange Under Compression

1. Analysis Method

1.1 Distortional Buckling

If the steel frame beams do not consider the deformation restraint by the upper concrete slabs, the members are freely deformed and twisted. This means that the total instability of the bending member occurs (lateral torsional buckling, steel standard, Section 6.2.1). Since both the steel beam and the concrete slabs are available as two independent structural components, the compatibility of deformation does not apply in this situation.

Lateral Torsional Buckling of Frame Beam Without Considering Concrete Slabs

However, if the frame beam of the steel structure is laterally supported by the concrete slabs, its stability usually does not need to be analyzed: According to GB 50017, Section 6.2.1, the beam's lateral-torsional buckling does not need to be designed if the concrete slabs are firmly connected to the compression flange of the beam so that the lateral displacement of the beam's compression flange is prevented. There are several ways in RFEM to model the composite effect of members and floor slabs, such as rib beams and surface model members. However, they represent integral structural components of concrete slabs in contrast to the explanations on frame beams above. Please find more detailed information on this subject in the corresponding articles in our Knowledge Base and in the Online Manuals.

Distortional Buckling of Frame Beam Considering Concrete Slabs

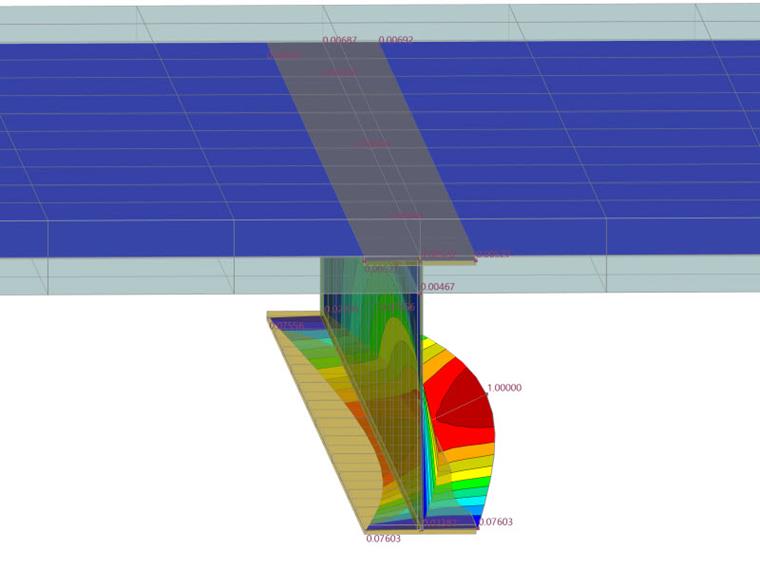
The image above shows the result of the FE calculation for the case that the top flange of the frame beam is supported. Thus, the bottom flange rotates about the center of the top flange. Therefore, according to [1] Section 6.2.7, the stability of the bottom flange must be checked.

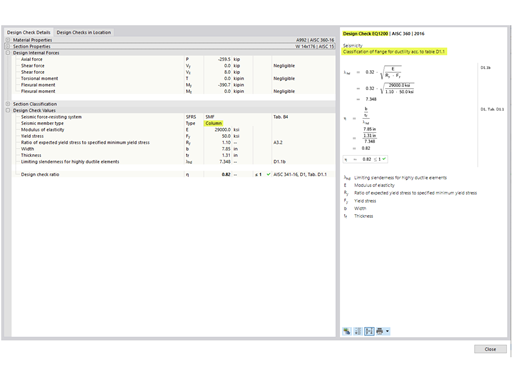
1.2 Design Check Procedure According to EC 3

The design method according to EC 3 [2], Sections 6.3.2.4, 6.3.5.2 and BB.2 differs from the GB steel construction standard. Structural components with laterally supported compression flanges may be considered as not prone to lateral-torsional buckling if specific conditions are met. For the design check regarding distortional buckling of a frame beam, the rotational stiffness and thus the torsional restraint of the bottom flange is checked first, as shown in the following image.

Lateral Support According to EC 3, 6.3.5.2 (Torsional Restraint)

1.3 Design Check of Distortional Buckling of Bottom Flange According to GB 50017

If the frame beam has a negative bending moment in the area near the support and a concrete slab is set upon the top flange, the stability calculation of the bottom beam flange must meet the following requirements according to Section 6.2.7 [1]:

(1) If λ_n,b ≤ 0.45, no design check is required for the bottom flange.

(2) If condition (1) is not met, the stability of the bottom flange must be calculated according to the following formula:

GB 50017, 6.2.7-1

$\frac{M_{x}}{φ_{d} \cdot W_{1 x} \cdot f} \leq 1.0$

GB 50017, 6.2.7-3

$λ_{n, b} = \sqrt{\frac{f_{y}}{σ_{c r}}}$

GB 50017, 6.2.7-4

$σ_{c r} = \frac{3.46 b_{1} t_{1}^{3} + h_{w} t_{w}^{3} (7.27 γ + 3.3) φ_{1}}{h_{w}^{2} (12 b_{1} \cdot t_{1} + 1.78 h_{w} t_{w})} E$

GB 50017, 6.2.7-5

$γ = \frac{b_{1}}{b_{w}} \sqrt{\frac{b_{1} t_{1}}{h_{w} t_{w}}}$

GB 50017, 6.2.7-6

$φ_{1} = \frac{1}{2} (\frac{5.436 γ h_{w}^{2}}{l^{2}} + \frac{l^{2}}{5.436 γ h_{w}^{2}})$

Where:

b₁ — width of compression flange (mm)
t₁ — thickness of compression flange (mm)
W_1x — gross cross-sectional modulus around strongest compressed fiber in plane of bending moment (mm3)
ψ_d — stability factor
λ_n,b — standardized limiting slenderness
σ_cr — critical stress for distortional buckling (N/mm2)
l — length between laterally supported points (mm)

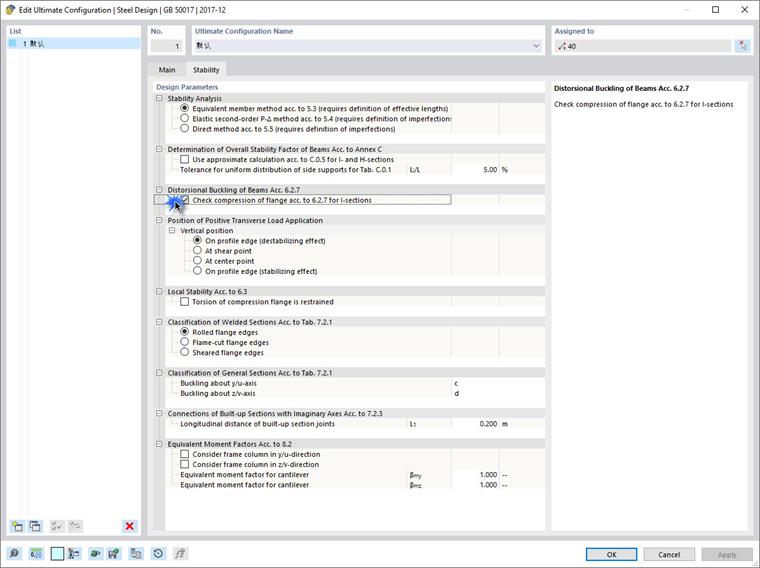
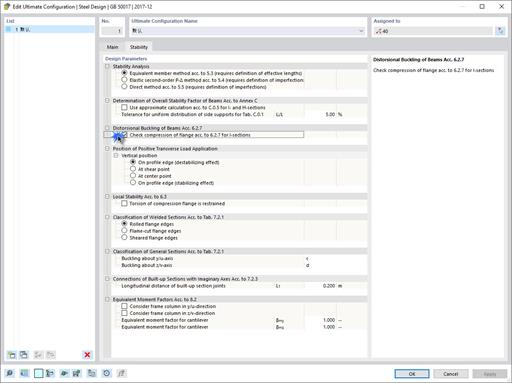
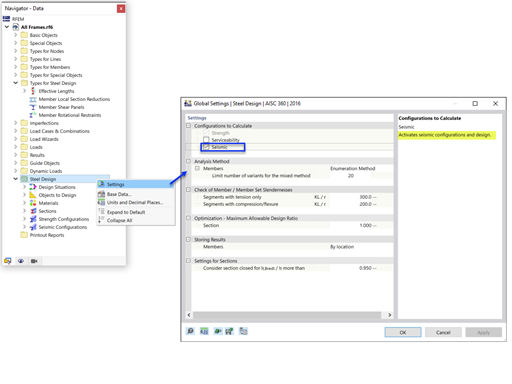
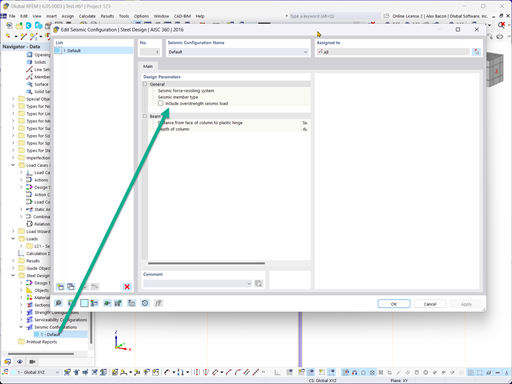
In RFEM, the corresponding function can be activated in the design configuration of the ultimate limit state setting.

Activating Design Check for Distortional Buckling of Bottom Flange

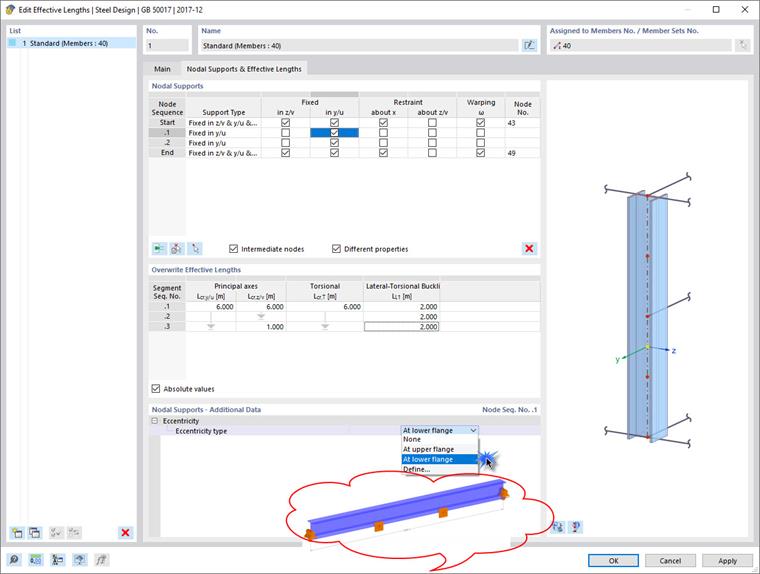
The lateral support points can be specified under the effective lengths.

Settings for Laterally Supported Bottom Flange Points

(1) After adding the intermediate nodes, the program automatically calculates the lateral support length in the negative moment zone of the frame beam. The other design lengths remain unchanged. The laterally supported distance is automatically taken into account.

(2) The specifications for load combinations and design situations remain unchanged.

(3) If the limiting slenderness is not exceeded, the design check result points out that the stability of the frame beam's bottom flange is not calculated. Otherwise, a detailed distortional buckling analysis is carried out to determine whether further laterally supported points are required.

(4) The laterally supported compression flanges still have to meet the requirements of the earthquake standard GB 50011, Chapter 8.3.3.

Design Check Details for Distorsional Buckling of Bottom Flange

2. Summary

In this article, the calculation procedure for designing the distortional buckling of the bottom flange was presented. The program checks whether the conditions of the torsional restraint are met by the lateral supports, or whether a further design check must be carried out in order to exclude distortional buckling. The design details are simple and understandable.

KB 001824 | Distortional Buckling Design of Bottom Flange of Steel Frame Beam According to GB

Model | GB Distortional Buckling - Surface Model

Model | Test GB Distortional Buckling of Member Section 01

Model | GB Distortional Buckling of Member Section 02

Author

Dipl.-Ing. (FH) Shaobin Ding, M.Sc. 丁少斌

Product Engineering & Customer Support

Mr. Ding is responsible for the product development and technical translation, and provides technical support to the Chinese customers.

Keywords

Steel Structures GB 50017 Distortional Buckling of Bottom Flange Torsional Restraint Frame Beam Negative Moment Section

Reference

[1]	Standard for design of steel structures
[2]	Eurocode 3: Design of steel structures - Part 1‑1: General rules and rules for buildings; EN 1993‑1‑1:2010‑12
[3]	GB 50017 Manual of steel sturcture design