5943x

001576

Dipl.-Ing. (BA) Sandy Matula

2019-06-12

Elastic-Plastic Cross-Section Design

The following article describes designing a two-span beam subjected to bending by means of the RF-/STEEL EC3 add-on module according to EN 1993-1-1. The global stability failure will be excluded due to sufficient stabilizing measures.

Structural System and Loading

Structure, Loading, Internal Forces

Girder Section HEA 600, S235

Design of a Cross-Section Class

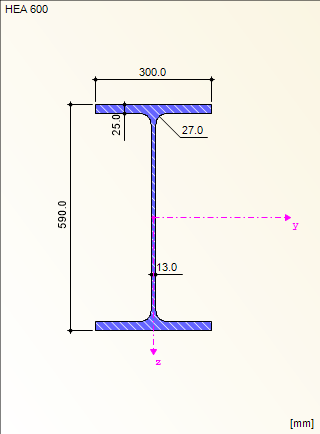
The area of the inner support of the two-span beam governs for designing the cross-section class and performing the cross-section design.

Design for the web (ψ = -1):
[1] Table 5.2, cross-section parts supported on two sides

\begin{array}{l} c = 590 - 2 \cdot (25 27) = 486 mm \\ existing \frac{c}{t_{w}} = \frac{486}{13} = 37.38 \\ limit \frac{c}{t_{w}} = 72 \cdot ε = 72 \cdot 1 = 72 > 37.38 \end{array}

Formula 1

The web thus fulfills the requirements of cross-section class 1.

Design for the bottom chord (ψ = 1):
[1] Table 5.2, cross-section parts supported on one side

\begin{array}{l} c = \frac{300 - (13 2 \cdot 27)}{2} = 116.5 mm \\ existing \frac{c}{t_{f}} = \frac{116.5}{25} = 4.66 < 9 \cdot ε = 9 \cdot 1 = 9 \end{array}

Formula 2

The chords thus fulfill the requirements of cross-section class 1. The cross-section has to be assigned to cross-section class 1.

Elastic-Plastic Cross-Section Design

\begin{array}{l} W_{pl, y} = 2 \cdot (30 \cdot 2.5 \cdot 28.25 27 \cdot 1.3 \cdot 16) = 5, 360 {cm}^{3} \\ M_{pl, y, Rd} = W_{pl, y} \cdot \frac{f_{y}}{γ_{M 0}} = 5, 360 \cdot \frac{23.5}{1} = 125, 960 kNcm = 1, 259.6 kNm \\ A_{v, z} = A - 2 \cdot b \cdot t_{f} (t_{w} 2 \cdot r) \cdot t_{f} = 226 - 2 \cdot 30 \cdot 2.5 (1.3 2 \cdot 2.7) \cdot 2.5 = 92.75 {cm}^{2} \\ V_{pl, Rd} = A_{v, z} \cdot \frac{f_{y}}{\sqrt{3} \cdot γ_{M 0}} = 92.75 \cdot \frac{23.5}{\sqrt{3} \cdot 1} = 1, 258.41 kN \end{array}

Formula 3

The cross-section design is performed for the cross-section of class 1. Above the inner support, the beam is subjected to bending and shear force; at the location of the maximum moment in the span, it is subjected only to bending. The influence of the M-V interaction is checked before determining the ultimate limit state of the structure. If V_Ed is not more than 0.5 ⋅ V_pl,Rd, the moment resistance is not required to be reduced according to [1], Section 6.2.8 (2).

\frac{V_{z, Ed}}{V_{pl, z, Rd}} = \frac{853.55}{1, 258.41} = 0.678 > 0.5

Formula 4

The moment resistance has to be reduced.

For I-cross-sections with the same flanges and uniaxial bending around the principal axis, the reduction of the design value of the plastic moment resistance due to the shear loading is allowed to be determined as follows:

\begin{array}{l} ρ = {(\frac{2 \cdot V_{Ed}}{V_{pl, Rd}} - 1)}^{2} = {(\frac{2 \cdot 853.55}{1, 258.41} - 1)}^{2} = 0.127 \\ A_{w} = h_{w} \cdot t_{w} = (59 - 2 \cdot 2.5) \cdot 1.3 = 70.2 cm \\ M_{pl, V, Rd} = (W_{pl, y} - \frac{ρ \cdot A_{w}^{2}}{4 \cdot t_{w}}) \cdot \frac{f_{y}}{γ_{M 0}} = (5, 360 - \frac{0.127 \cdot 70 . 2^{2}}{4 \cdot 1.3}) \cdot \frac{23.5}{1 \cdot 100} = 1, 231.32 kNm \\ M_{Ed} < M_{pl, V, Rd} \to 1, 068.36 < 1, 231.32 kNm \end{array}

Formula 5

Author

Ms. Matula provides technical support for our customers.

Links

Structural Analysis Software for Steel Structures

References

European Committee for Standardization (CEN). (2010). Eurocode 3: Design of Steel Structures – Part 1‑1: General Rules and Rules for Buildings, EN 1993‑1‑1:2010‑12. Berlin: Beuth Verlag GmbH.
Dlubal Software. (2020). Manual RF-/STEEL EC3. Tiefenbach: Dlubal Software.
Albert, A. (2018). Schneider – Bautabellen für Ingenieure mit Berechnungshinweisen und Beispielen (23rd ed.). Cologne: Bundesanzeiger.

;