Answer:
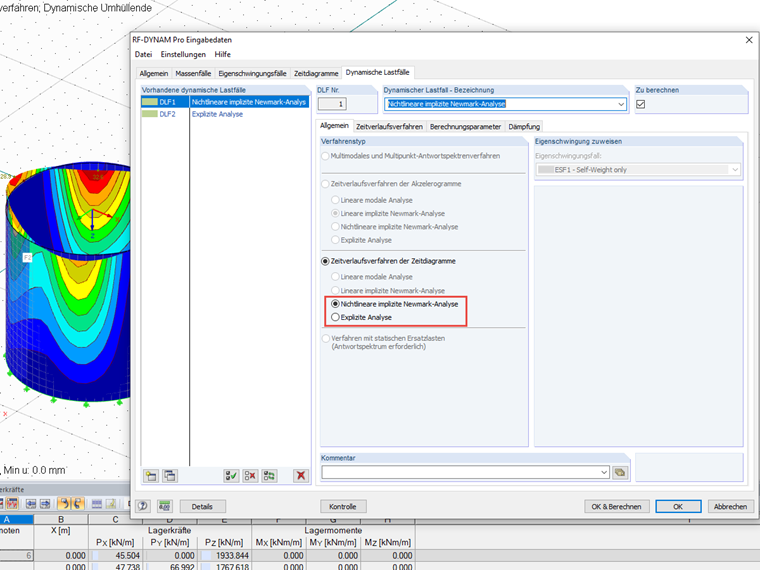
In the RF-DYNAM Pro - Nonlinear Time History Analysis add-on module, two different methods are available for nonlinear dynamic analysis: the explicit Central Difference Method and the implicit Newmark analysis of the mean acceleration.
Nonlinear Implicit Newmark Analysis
The nonlinear Newmark analysis is an implicit method that solves the unknown values at the time i+1 in relation to the values of the time i and i+1. This method is numerically stable, regardless of the selected time step length. This is one reason why this method is usually preferred for dynamic loads acting over a longer period of time.
Explicit Analysis
The explicit analysis is unique, as it solves values independently of the unknown values at the time i+1. However, this is the reason that the explicit analysis is only stable if the selected time step is smaller than a certain, critical time step. The calculation time of a single time step is very short, but numerous; very fine time steps may be required to obtain a result at all.
Therefore, the explicit solver is preferred if you have to select very fine time steps anyway in order to obtain a useful (converging) result. This is the case, for example, for very short-term and abruptly variable loads, such as impact loads or explosion loads.
Conclusion
In summary, both methods are "only" numerically stable in the nonlinear area, while the implicit Newmark analysis is still more stable than the Central Difference Method in most cases.
However, it should be noted that in the case of a Newmark analysis, it is necessary to pay attention to a sufficiently small time step in order to achieve exact results. Which time step should be selected in a dynamic calculation is explained in detail in FAQ 2655.
More information, in particular about the critical time step in the explicit analysis, can be found in the RF‑DYNAM Pro manual.
